Continuous Fractional Derivative of Exponential Function

Written on December 8th, 2017 by Kevin Ahrendt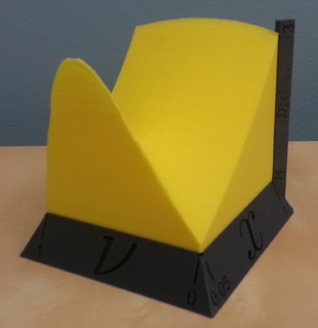

Description:

This model is a three dimensional graph \(e^x\), with one axis representing the Riemann-Liouville fractional derivative order ranging from 0 to 1. The x-axis ranges from 0.05 to 1; the fractional derivative of \(e^x\) approaches infinity as x approaches 0, hence the axis starts at 0.05 avoid the asymptote. The Riemann-Liouville fractional derivative is continuous with the respect to the order of the derivative, so this graph is nice and smooth.

Design process:

I used Mathematica to generate the graph using the included Mathematica code. Then I used Slic3r to scale the Mathematica output to be \(80\times80\times80\) \(\text{mm}^3\) (as well as let it correct some errors in the stl file). Finally, I used Fusion 360 to design the stand.

Post-print finishing:

If desired, use a few drops of super glue to attach the surface to the stand.

Files